






I derived this formula (see pp 2-6 of the attached document) on a rainy Saturday morning in Indianapolis in the Fall of 1982 while doing a homework exercise for Dr Greenleaf's Investment Management Course. I gave the formula to Dr Greenleaf the following week during our period break. The professor was never late returning to class but that day we waited 5-10 minutes before he got back.
"It ought not work, Mr. Joules, but it does!" The professor had apparently tried several examples, certain that the formula would break, as he had not seen my derivation and had assumed that I had just taken a wild swing at creating a simple closed-form equation for Macaulay's Duration of a Bond. The best-known up to that point was probably the formula by Boquist, listed at the bottom of the References section of the attached document.
Later, I gave my derivation to the professor and asked if we could publish it together as I had no idea, and did not have the time to learn, how to go about getting it published on my own. While seeking interested publications Professor Greenleaf came across Professors John Caks and William Lane, who apparently had arrived at the same formula through an approach different than mine. They agreed to credit all four names regardless of which article got accepted first by a publisher. Their article was accepted by The Journal of Financial Research. It was published in the Fall of 1985.
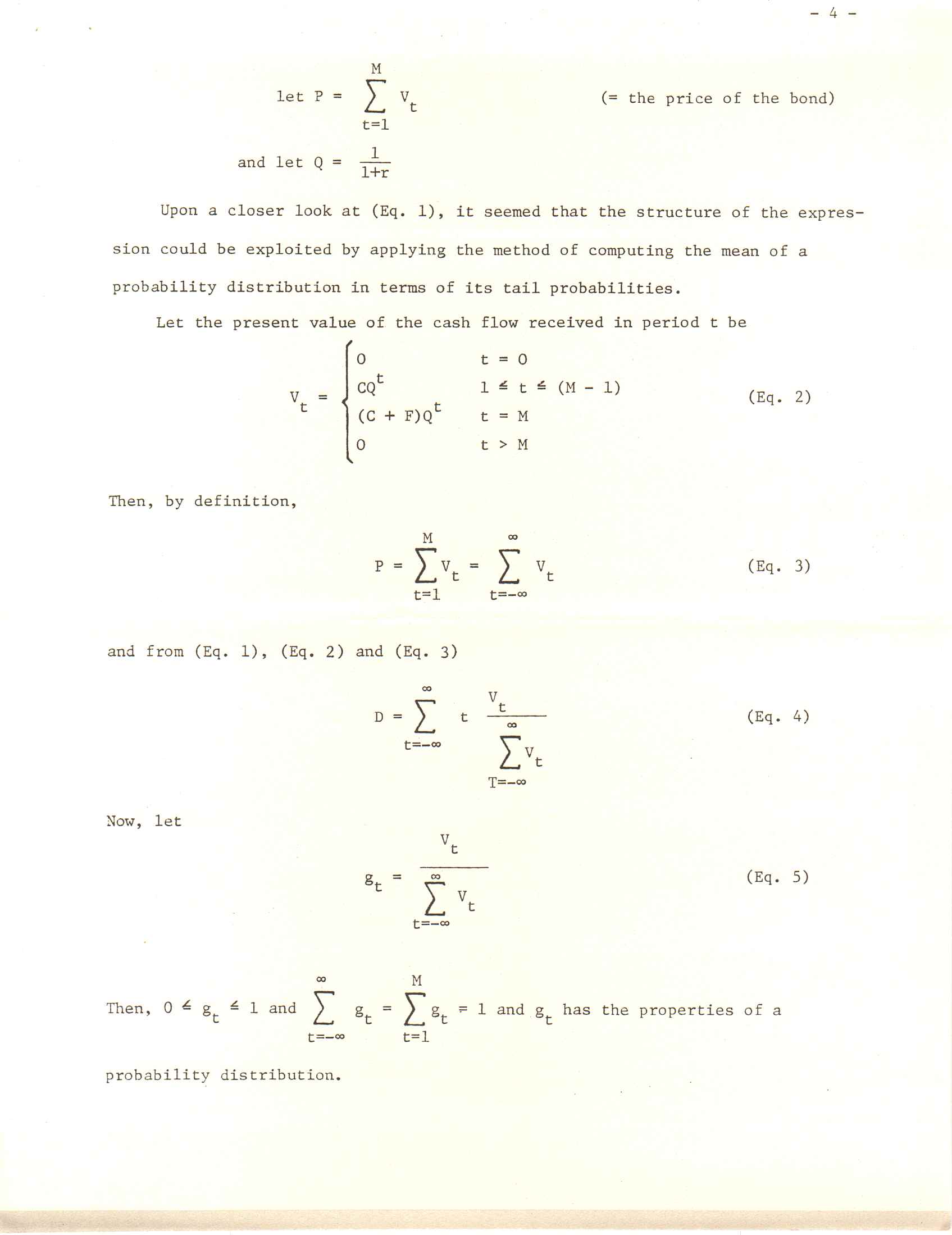
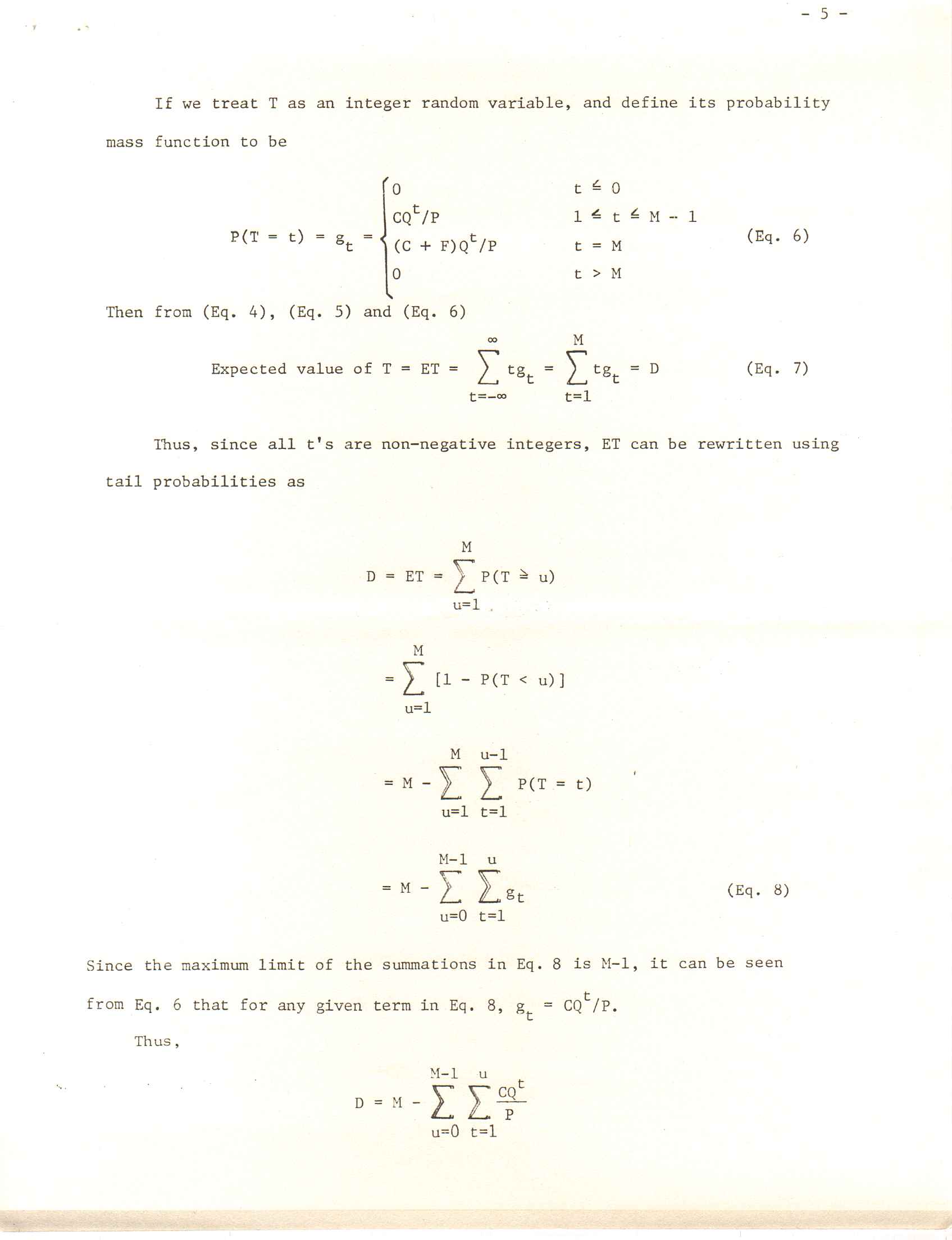
My approach in the attached document does not use any financial concepts to morph the original mathematical summation series for Duration into a simple closed form equation. Instead, I exploited the mathematical structure of Macaulay's expression for Duration, demonstrating that the series had the same mathematical properties as that of an expression for calculating the expected value of a discrete random variable, using tail probabilities.
It seemed at the time that the financial publications community could not see how probability modeling could be applied for collapsing an unwieldy mathematical expression of a deterministic financial concept into a simple closed-form equation.








